In mathematics, exponents are a fundamental concept represented by symbols or figures that indicate how many times a quantity should be multiplied by itself. Let’s explore this fascinating topic further!
Understanding Exponents:
Exponential functions are typically of the form: f(x) = ax, where a is referred to as the base, and x is a real number.
It’s important to note that there’s a distinction between a typical function in the form xa, where x is the variable and a is a fixed number, and an exponential function where ax, where a is the variable, and x is a fixed number.
Exploring the Function:
Let’s work with the function f(x) = 2x for illustrative purposes. This example will help us grasp the concept.

Graphical Insight:
- When x = -2, we find that f(-2) = 0.25. The value 0.25 is close to zero, and the graph nearly touches the x-axis.
- At x = -1, f(-1) = 0.5. Here, 0.25 < 0.5, and the graph rises. At x = 0, f(0) = 1, and the graph crosses the y-axis, continuing to rise.
- For x = 1, we have f(1) = 2. Notably, 0.25 < 0.5 < 1 < 2, indicating rapid growth in the graph.
- Finally, at x = 2, we find that f(2) = 4.
In summary, as the value of x increases, the value of y also increases.
Solving Exponential Equations:
Let’s work through examples to solve exponential equations:
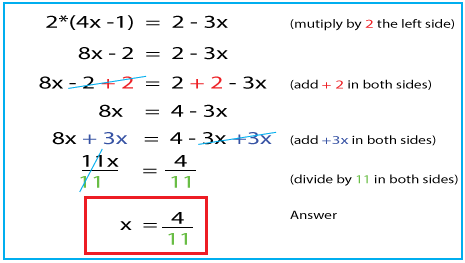
Solve for: 52*(4x – 1) = 5(2 – 3x)
Solution:
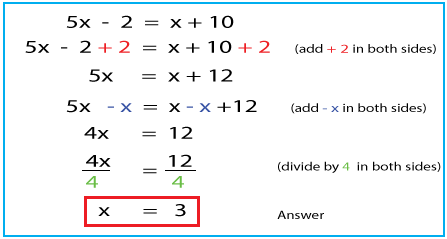
Solve for: 3(5x – 2) = 3(x + 10)
Solution:
Explore more examples and enhance your understanding of exponential functions by downloading our free courses.


