HISTORY: Derivation is very important in mathematics especially in calculus.
Appeared by the end of the 16th century, this concept was developed during the 17th century, by a great German philosopher and scientist named Gottfried Wilhem Leibniz (1646-1716) and also by a great English scientist and inventor named Sir Isaac Newton(1642-1727).
Note the German scientist Gottfried Wilhem Leibniz (1646-1716)introduce the following notation: df/dx .
Note the French mathematician Joseph Louis Lagrange (1736-1813) introduce the “prime” notation f ‘(x).
LISTS OF TRIGONOMETRY DERIVATIVES:
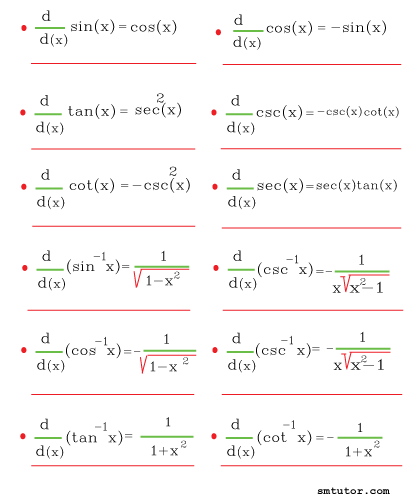
List of some mathematic formulas here: Derivative, Integral, and more…
EXAMPLE 1: Derivative [cos( u ) ]‘ = u ‘ * [– sin(u)]
| Find the derivative of f(x) = [cos(4x)]
Solution: f‘(x) = (4x)‘ * [–sin(4x) ]‘ f‘(x) = 4 * [–sin(4x) ] f‘(x) = – 4 sin(4x) |
EXAMPLE 2: (u * v )‘ = u‘ v + v‘ u
| Find the derivative of f(x) = 2x * cos(3x)
Solution: f‘(x) = [ 2 x ]‘. cos(3x) + [cos(3x)]‘. 2 x f‘(x) = 2 [cos(3x)] + 2x .[ cos(3x)]‘ f‘(x) = 2 cos(3x) + 2x .[(3x)‘. -sin(3x)] f‘(x) = 2 cos(3x) + 2x .[(3) . -sin(3x)] f‘(x) = 2 cos(3x) + 6x . -sin(3x)] f‘(x) = 2 cos(3x) – 6xsin(3x) |
