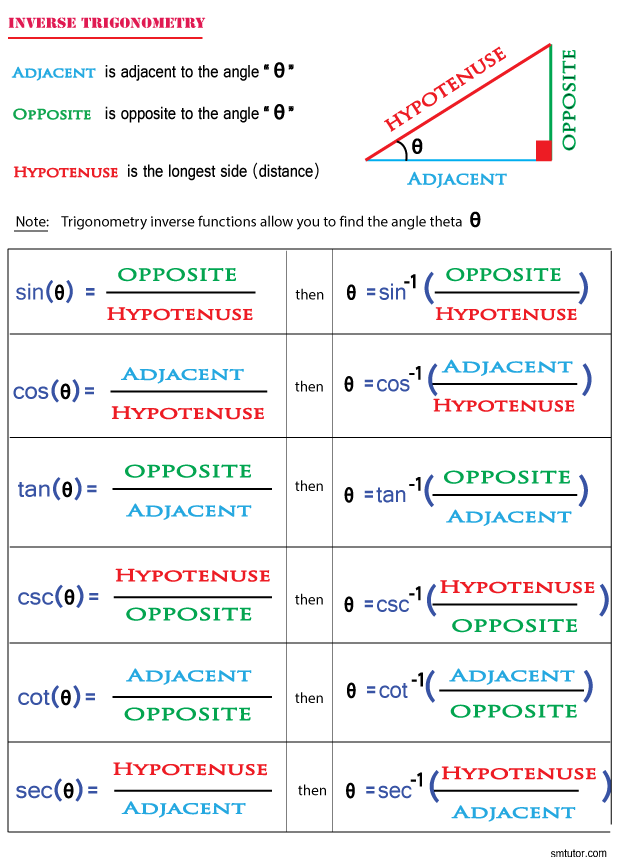
Definition: A trigonometry function is defined as a function with an angle. The angle is often called (theta noted ” θ “) or ( phi noted “ φ “). Also, You should note that the angles can be determined in Degrees(example: 300, 900) or in Radians(example: π/2 rad, π/3 rad).
The Inverse trigonometry functions are the inverse functions of the trigonometry function. Below is the example.
- Inverse Sine function : Example: sin-1(θ)
- Inverse Cosine function : Example: cos-1(θ)
- Inverse Tangent function : Example: tan-1(θ)
- Inverse Cosecant function : Example: csc-1(θ)
- Inverse Secant function : Example: sec-1(θ)
- Inverse Cotangent function : Example: cot-1(θ)
 |
Calculation Example
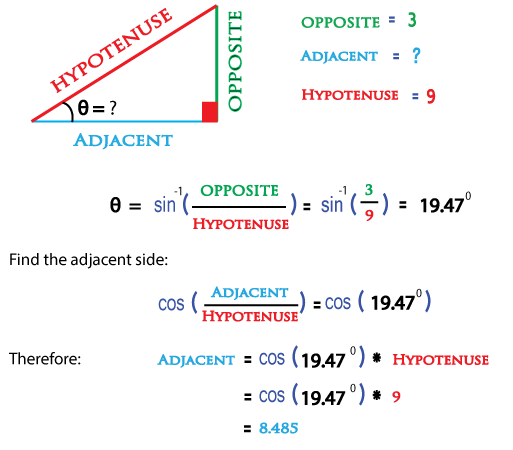
Cases1. Solving for an angle knowing three (3) sides: Hypotenuse, Adjacent, and Opposite.
a) Example 1: What is the angle θ if hypotenuse = 9, adjacent = ? and opp0site = 3.
Solve: For θ ? and what is the angle of the adjacent side?
Solution:
In this example, we will be looking at the right triangle as the reference in order to determine the value of each specific angle. See below ⇓
 |
See more
All Trigonometry Functions and Inverse Trigonometry Functions Domain and Range by downloading the full infographics Table.


