Definition: Understanding limits is a fundamental concept in mathematics, and it can seem daunting at first. In this chapter, we’ll break down the idea of limits to make it accessible and clear. We’ll begin by exploring limits at infinity (+ ∞ and – ∞).
PART 1: Infinite Limits
| lim x = + ∞ |
| x-> +∞ |
Infinite Limit functions in – ∞:
| lim x = – ∞ |
| x-> – ∞ |
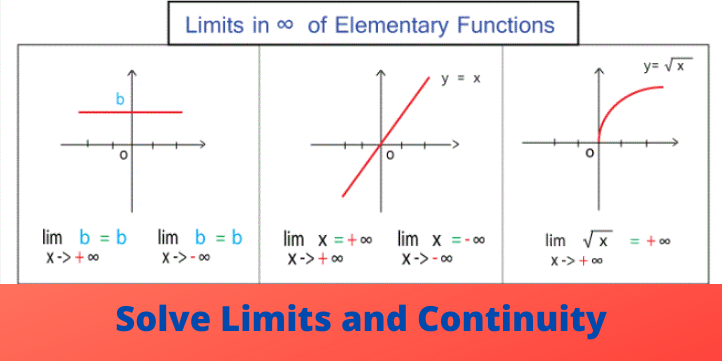
PART 2: Finite Limits
Now, let’s dive into finite limits. When a function has a finite limit at a point a0, it means the function approaches a specific value as it gets closer to that point. This unique limit is a defining feature of mathematical functions.
| lim f(x) = f(a0) |
| x-> a0 |
Understanding limits has practical applications beyond mathematics. It plays a crucial role in various fields, including physics, engineering, and computer science. Engineers use limits to analyze systems’ behaviors, and physicists rely on limits to describe the behavior of particles at the quantum level. Even in computer science, algorithms often involve the concept of limits for efficiency and accuracy.
Ready to explore limits further? Discover a wealth of examples and in-depth explanations by downloading our free courses. This valuable resource will help you master limits, create informative content, and adhere to HTML standards while optimizing for SEO.


