Welcome to the Fascinating World of Quadratic Equations! 
In the realm of mathematics, quadratic equations are like hidden gems, waiting to be discovered and cherished. Join us on this exciting journey of exploration!
Quadratic equations are mathematical marvels defined by the power of squares. The term “quadratic” derives from the word “quad” meaning “square.” They take the form of ax2 + bx + C, where a ≠ 0. Let’s dive deeper into this fascinating world!
- Behold, a Quadratic Function: Example: x2 + 4x + 3
In its standard form, a quadratic equation is represented as:
ax2 + bx + c = 0
Note that a, b, and c have their unique values. The degree of the equation is 2, and the unknown variable is represented by X.
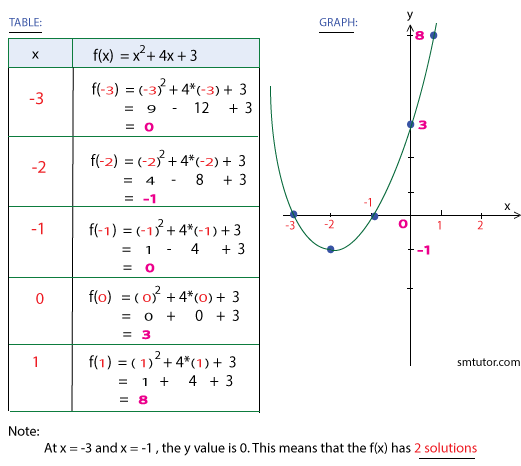 |
How to Solve Quadratic Equations in 3 Intriguing Cases:
Let’s unravel the mysteries of quadratic equations through three captivating cases:
Case 1: Solving by Completing the Square
In this case, we’ll delve into the art of completing the square to conquer quadratic equations.
Case 2: Solving by Factoring the Quadratic
We’ll explore the fascinating technique of factoring quadratic equations in this case.
Case 3: Solving by using the Quadratic Formula
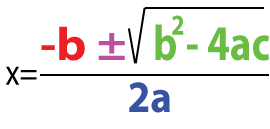
Discover the power of the quadratic formula to solve quadratic equations with precision.
Solving a Quadratic Equation:
Let’s dive into solving quadratic equations through two fascinating examples:
a) Example 1: Solve the following Quadratic Equation using the Quadratic Formula:
x2 + 6x + 8 = 0
Solution:
b) Example 2: Solve the following Quadratic Equation by Factoring:
x2 + 4x + 3
Master the art of quadratic equations by exploring more examples and courses:
Download the free courses
Congratulations, you’ve now unlocked the magic of quadratic equations! Keep exploring and conquering more mathematical wonders!






